

SOLVING SEQUENCES PROFESSIONAL
Tutors, instructors, experts, educators, and other professionals on the platform are independent contractors, who use their own styles, methods, and materials and create their own lesson plans based upon their experience, professional judgment, and the learners with whom they engage. Varsity Tutors connects learners with a variety of experts and professionals. Varsity Tutors does not have affiliation with universities mentioned on its website. Media outlet trademarks are owned by the respective media outlets and are not affiliated with Varsity Tutors.Īward-Winning claim based on CBS Local and Houston Press awards. Next two terms are \(,\,\,\_\_\,\,\,\_\_\) On the top (numerator), the next term is 1 more than the previous one, and the bottom (denominator), the next term is the previous term multiplied by 2.Names of standardized tests are owned by the trademark holders and are not affiliated with Varsity Tutors LLC.Ĥ.9/5.0 Satisfaction Rating based upon cumulative historical session ratings through 12/31/20. For example, try to find the next few terms in the following sequences:ġ, 8, 27, _, _ Every term is cubed. You may have heard the term inductive reasoning, which is reasoning based on patterns, say from a sequence (as opposed to deductive reasoning, which is reasoning from rules or definitions).
SOLVING SEQUENCES SERIES
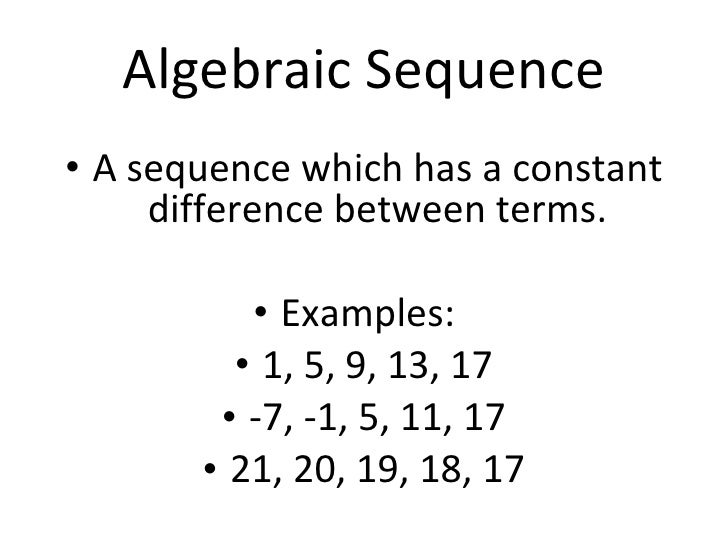
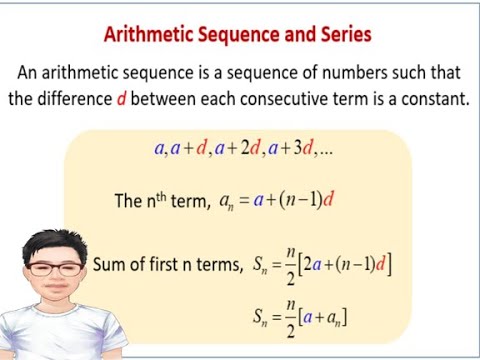
Sequences are the list of these items, separated by commas, and series are the sum of the terms of a sequence (if that sum makes sense it wouldn’t make sense for months of the year). Each of these numbers or expressions are called a term or an elements of the sequence. Sequences are basically just numbers or expressions in a row that make up some sort of a pattern for example, January, February, March, …, December is a sequence that represents the months of a year. Note that Limits of Sequences are discussed here in the Limits and Continuity section. Introduction to Sequences and Series Arithmetic Series Summary of Formulas for Sequences and Series Summation Notation Sequences and Series Definitions Geometric Series Explicit Formulas Versus Recursive Formulas Finite Geometric Series Arithmetic Sequences Infinite Geometric Series Geometric Sequences Applications of Sequences and Series Writing Formulas Sequences and Sums on the Graphing Calculator More Practice Applications of Integration: Area and Volume.Exponential and Logarithmic Integration.Riemann Sums and Area by Limit Definition.Differential Equations and Slope Fields.Antiderivatives and Indefinite Integration, including Trig.Derivatives and Integrals of Inverse Trig Functions.Exponential and Logarithmic Differentiation.Differentials, Linear Approximation, Error Propagation.Curve Sketching, Rolle’s Theorem, Mean Value Theorem.Implicit Differentiation and Related Rates Sequences Sequence Type: Linear Quadratic Cubic Geometric Negative First Coefficient: Question Type: Generate Sequence Next 2 Terms nth Term Find Term Use.Equation of the Tangent Line, Rates of Change.Differential Calculus Quick Study Guide.

Solving Quadratics, Factoring, Completing Square.Introduction to Multiplying Polynomials.Scatter Plots, Correlation, and Regression.Algebraic Functions, including Domain and Range.Systems of Linear Equations and Word Problems.Introduction to the Graphing Display Calculator (GDC).Direct, Inverse, Joint and Combined Variation.Coordinate System, Graphing Lines, Inequalities.Types of Numbers and Algebraic Properties.Introduction to Statistics and Probability.Powers, Exponents, Radicals, Scientific Notation.


 0 kommentar(er)
0 kommentar(er)
